Big Ideas Little Pictures

Sketchplanations in a book! I think you'll love Big Ideas Little Pictures

Sketchplanations podcast photo of Rob Bell, Tom Pellereau and Jono Hey

Prefer to listen?
Try the podcast

Like Sketchplanations?
Support me on Patreon

The haversine formula

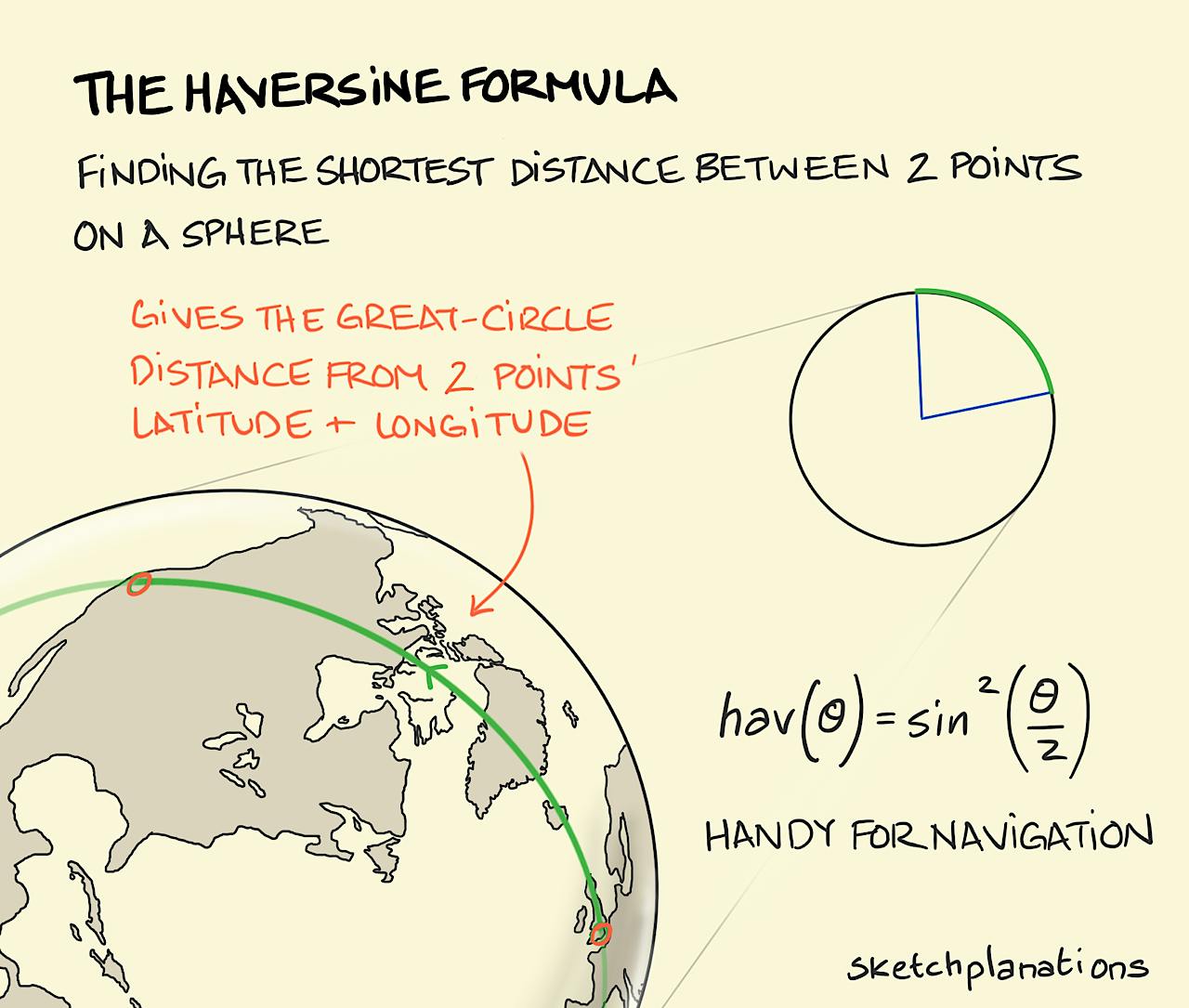
The Haversine Formula illustration: a portion of the earth's globe is shown with a green arc drawn between the cities of London, UK and Seattle, WA. The mathematical Haversine formula takes into account the curvature of the earth's surface when calculating the true distance between two points on a curved surface.

It might seem straightforward to pull out a map and measure the distance between two distant points, however, the larger the distance the bigger the distortion caused by traveling on the curved surface of the Earth as opposed to flat 2D space. So while the distance you measure to your neighbouring town won't be too bad, if you're measuring between London and Rio the curvature of the Earth will make a big difference to the distance that you'll travel. To help figure out the correct distance there's the haversine formula.

The haversine formula allows you to calculate the shortest distance between two points on a sphere using their latitudes and longitudes — this will be the arc between them on the great circle that includes both points. A great circle is a circle on a sphere with the same centre as the sphere, like the Equator. The haversine formula isn't perfect in practice, as Earth isn't a perfect sphere — see the 3 tallest mountains.

It was invented around two hundred years ago, together with tables to speed any calculations, to help sailors navigate.

Also see, the Mercator projection

Published 18 Oct 2020

A weekly sketch by email

Learn something new in a sketch each Sunday

Sketchplanations in a book! I think you'll love Big Ideas Little Pictures

Prefer to listen?
Try the podcast

Like Sketchplanations?
Support me on Patreon

Keep exploring

What is parallax explanation - how parallax works showing different layers moving at different speeds

The Village Venus effect example, a term from Edward De Bono, with a fish declaring that another fish in the fish bowl is the most beautiful fish in the whole world

The Village Venus Effect

The 3 Tallest Mountains illustration: on the left, Mount Everest is measured from sea level. In the centre, Mauna Kea is measured from its base (well below sea level) to its summit. On the right, Chimborazo is located near the equator where the earth bulges making its summit the furthest from the centre of the earth (and hence closest to the sun).

The 3 tallest mountains

Tax Freedom Day illustration: a year-long scale from January to December shows how long into the year it takes for different countries to cover the bill for public services through income tax. After that point, the money you earn is for you to spend however you like.

Tax freedom day

The automation paradox explanation, or paradox of automation, summary with a hiker choosing their phone in place of a physical map and then getting lost in a landscape when there's no signal

The Automation Paradox

What is Naismith's Rule for calculating walking time in the mountains example explained with a mountain ridge with hikers and lines for the horizontal distance and time and ascent and time

Naismith's Rule for mountain hiking time

Parallax is the change in the apparent position of objects from different viewpoints. For example, from one viewpoint, a nearby house may appear in front of a hill, and from another, it may be in front of a lake. Objects at different distances appear to move by different amounts. As you move between viewpoints, say when driving along a road, nearby objects move past you quickly, while distant objects appear to move by slowly. This difference in motion from near to far objects helps us determine how far objects are away. I remember learning parallax from video games, like playing Sonic the Hedgehog on the old Sega Mega Drive. As the character moves in the foreground, elements at different distances in the background move progressively slower, creating a sense of depth. Saturation and contrast change at with distance also, known as atmospheric perspective. I also enjoy observing parallax while looking out of a train window; nearby hedges and trees race by, buildings in the middle distance move more slowly, and distant hills or mountains barely seem to change. It's fascinating how our brains interpret this relative movement to judge distances. One of the more intriguing applications of parallax is stellar parallax in astronomy. The basic geometry of parallax allows us to measure the distance of (relatively) nearby stars by observing relative shifts against the background of distant stars. Here's how it works: we observe the stars from different points in our orbit around the Sun. As a result, the stars appear to move relative to each other. By measuring this apparent shift, we can calculate the distance of the stars. Also see: Redshift The Doppler effect Atmospheric perspective Two-point perspective Pace layers Time hierarchy…Parallax is the change in the apparent position of objects from different viewpoints. For example, from one viewpoint, a nearby house may appear in front of a hill, and from another, it may be in front of a lake. Objects at different distances appear to move by different amounts. As you move between viewpoints, say when driving along a road, nearby objects move past you quickly, while distant objects appear to move by slowly. This difference in motion from near to far objects helps us determine how far objects are away. I remember learning parallax from video games, like playing Sonic the Hedgehog on the old Sega Mega Drive. As the character moves in the foreground, elements at different distances in the background move progressively slower, creating a sense of depth. Saturation and contrast change at with distance also, known as atmospheric perspective. I also enjoy observing parallax while looking out of a train window; nearby hedges and trees race by, buildings in the middle distance move more slowly, and distant hills or mountains barely seem to change. It's fascinating how our brains interpret this relative movement to judge distances. One of the more intriguing applications of parallax is stellar parallax in astronomy. The basic geometry of parallax allows us to measure the distance of (relatively) nearby stars by observing relative shifts against the background of distant stars. Here's how it works: we observe the stars from different points in our orbit around the Sun. As a result, the stars appear to move relative to each other. By measuring this apparent shift, we can calculate the distance of the stars. Also see: Redshift The Doppler effect Atmospheric perspective Two-point perspective Pace layers Time hierarchy

More on these topics

…

…

…

…

…

…

…

…

…

…

…

Buy Me A Coffee